I'm new to skeptics SE, but, as I earn my living by analysing data, I thought I'd give it a try. My analysis is based on the raw data linked by Oddthinking (+1).
The claim in the question:
One in four victims of intimate partner homicide in Australia are male
is true, as far as the data can tell:
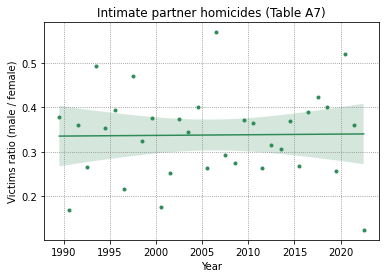
Per every female victim there are, on average, 0.337 male victims. Or, in terms of risk ratios, 25.2% of all victims are male. The ratio is highly stable over the years (p > 0.9).
This stability is in line with what Oddthinking found about another claim:
there has been a 75% reduction in the rate of female victims and but only a 30% reduction in the rate of male victims.
Both victim rates fall over time with almost the same speed, relative to the initial rate:
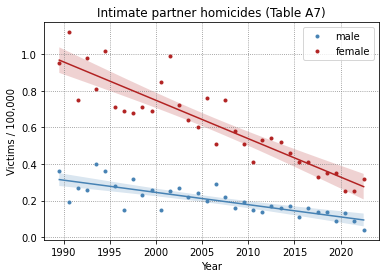
Edit in response to comments:
As we are talking about rates and the numbers of victims can never be negative, linear model is not the most appropriate one. It is simple and sufficient enough to answer the question (plus, I can draw the nice confidence band), but, strictly speaking, a Poisson model is better (it is a generalised linear model, where we assume the exponent to behave linearly with time).
For male victims we obtain:
Results: Poisson
==============================================================
Model: Poisson Method: MLE
Dependent Variable: rMale Pseudo R-squared: 0.024
Date: 2024-05-22 09:34 AIC: 33.6676
No. Observations: 34 BIC: 36.7203
Df Model: 1 Log-Likelihood: -14.834
Df Residuals: 32 LL-Null: -15.195
Converged: 1.0000 LLR p-value: 0.39544
No. Iterations: 5.0000 Scale: 1.0000
---------------------------------------------------------------
Coef. Std.Err. z P>|z| [0.025 0.975]
---------------------------------------------------------------
Intercept -1.4390 0.3958 -3.6358 0.0003 -2.2148 -0.6633
year -0.0334 0.0399 -0.8365 0.4029 -0.1115 0.0448
==============================================================
and for female:
Results: Poisson
==============================================================
Model: Poisson Method: MLE
Dependent Variable: rFemale Pseudo R-squared: 0.041
Date: 2024-05-22 09:34 AIC: 58.0778
No. Observations: 34 BIC: 61.1305
Df Model: 1 Log-Likelihood: -27.039
Df Residuals: 32 LL-Null: -28.206
Converged: 1.0000 LLR p-value: 0.12653
No. Iterations: 5.0000 Scale: 1.0000
---------------------------------------------------------------
Coef. Std.Err. z P>|z| [0.025 0.975]
---------------------------------------------------------------
Intercept -0.3245 0.2265 -1.4331 0.1518 -0.7684 0.1193
year -0.0344 0.0229 -1.5025 0.1330 -0.0794 0.0105
==============================================================
As you can see, both rates decrease with almost the same yearly rate, exp(-0.334) = 0.967 for males and exp(-0.344) = 0.966 for females. exp(-0.3245) = 0.72 is the modelled rate for women in my reference year, the year 2000, and exp(-1.4390) = 0.24 for men:
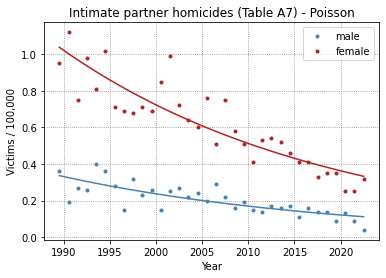
The ratio of the two rates is 3.05, i.e. for every three female victims there is almost exactly one male victim. That answers the original question. Anyone having questions regarding statistical methodology, please post them on CrossValidated.

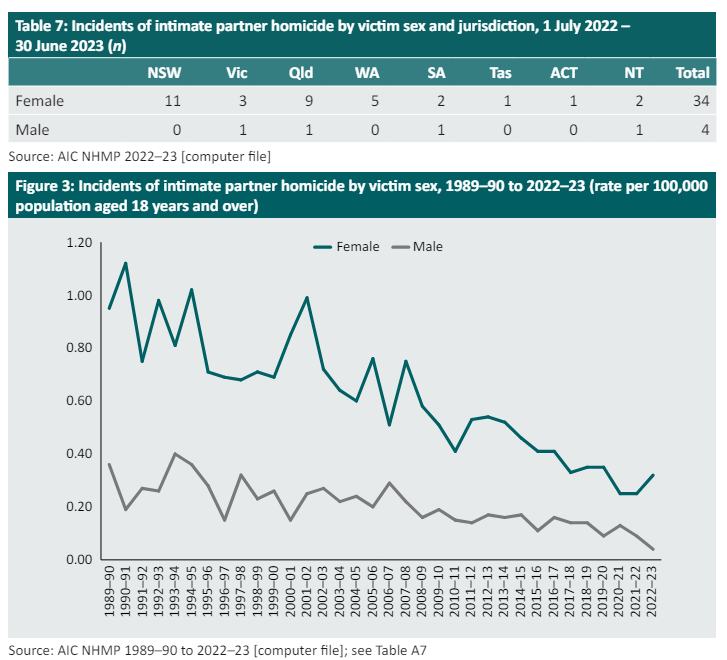



&t=807to the back of your link.