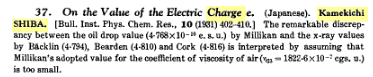No.
A much more accurate account is in THE CHARGE OF THE ELECTRON (1935):
In 1929 Prof. R. T. Birge (reference 1) published an acutely critical and masterly survey of our knowledge of the fundamental physical constants. It was a very timely summary, and it undoubtedly-if we may borrow from the vocabulary of
another trade-did much to promote a desirable "constants-consciousness ” in the
general body of physicists. This especially applies to two of the atomic constants,
the charge e and the specific charge e/m of the electron.
At the time of Birge’s report, there were two distinct, and apparently equally
well authenticated, values of e/m. Direct deflection methods gave 1.769 x 10^7,
while spectroscopic methods gave 1.761 x 10^7 abs. e.m.u./ gm. As the maximum
error admitted in each case was only of order 1 part in 1000, the relatively large
difference between the two values was more than a little disturbing. On the other
hand, Millikan’s value of e (only slightly modified in revisions of the calculations,
made by Birge(1) and by Millikan(2) himself) was generally accepted as accurate
to within 1 part in 1000. In fact it seemed very likely that the electronic charge
lay somewhere between 4.768 and 4.772 x 10 ^ - 10 e.s.u. It is significant of the
authority attaching to Millikan’s work that it was considered necessary to correct
his values for the small difference between absolute and international electrical
units and for a small change in the accepted value of the velocity of light.
The situation has oddly changed since 1929, the spectroscopic and deflection
values of e/m now being in excellent agreement at something very near to 1758 x 10^7.
There are, however, now in the field two values of e which differ by more than
7 parts in 1000; they bear in fact to one another almost exactly the celebrated and
possibly significant ratio 136/137(3). The first of these is Millikan’s, the second is
the value deduced from absolute X-ray wave-lengths by a method which was only
beginning to be fully exploited at the time of Birge’s first paper.
where reference 1 is Probable Values of the General Physical Constants (1929) reference 2 is Millikan's THE MOST PROBABLE 1930 VALUES OF THE
ELECTRON AND RELATED CONSTANTS (1930)
In other words, by 1929 there was data by a different technique that contradicted Millikan's value.
For further information see:
Note on the Value of the Electric Charge (1929)
Absolute Wave-Lengths of the Copper and Chromium K-Series (1931) (finds the e is 4.806 × 10^−10 e.s.u., above the currently accepted/defined value of 4.8032 × 10^−10 e.s.u.)
Viscosity of Air and the Electronic Charge (1935).
The charge of the electron (1937) (a follow up to the 1935 article with the same title)
The Atomic Constants A Revaluation and an Analysis of the Discrepancy (1939)
and see:
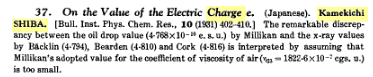
where the values found by both Bearden and Cork are above the current value.
So in 1931 it was first realized that Millikan's 1930 value disagreed with X-ray technique values due to Millikan's use of an inaccurate viscosity of air value. And this was generally accepted by the 1935-1939 time frame.
In conclusion, there was no hesitation to report correct values in the primary (experimental) literature, at most there was an initial reluctance in the secondary (review/commentary) literature to accept that the values by the new X-ray technique were correct.