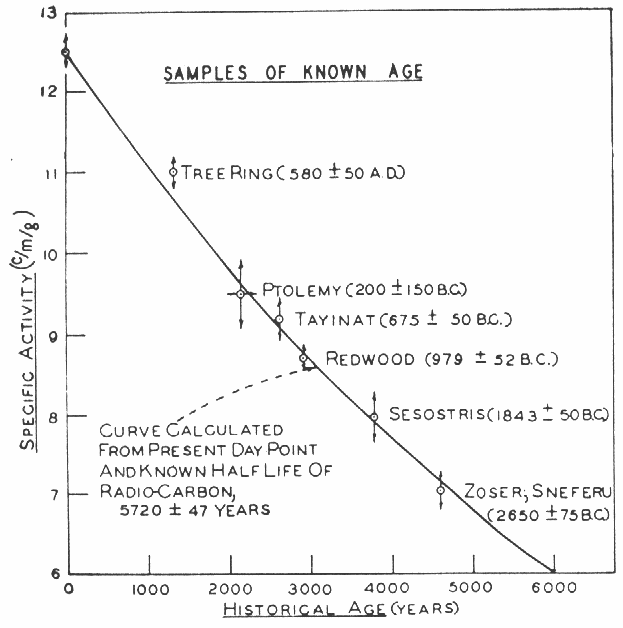
Before addressing the title of the question, I will first address the premise of the question:
Creationists make the claim that a global flood has messed up the precision of radiocarbon dating and hence, it cannot be relied upon. But if it is consistently cross-referenced by other methods, then the claim doesn't quite work.
If those same creationists claim that the flood was supernatural, and that which is supernatural isn't within the realm of natural, they could also argue that any other methods it would be cross referenced with would either be
- also affected by the supernatural flood or
- supernaturally placed there in some other way.
The same way they would argue a supernatural flood caused x to be different than the accepted laws of nature, they could just as easily argue that either the same, or similar, or different supernatural events caused any other cross referenced methods to be the way they are.
Their arguments aren't based on the scientific method, so using physical, natural evidence wouldn't apply to the the same way it would apply to a scientist, for example.
Now, to the question: Are results of carbon dating cross referenced by other methods?
The short answer is Yes
An article from nature.com details some examples of recent tree ring calibrations which are said to have changed the previously assumed dates (that were reached through radioactive dating) by different amounts, for example:
For the first time in seven years, the technique is due to be recalibrated using a slew of new data from around the world. The result could have implications for the estimated ages of many finds — such as Siberia’s oldest modern human fossils, which according to the latest calibrations are 1,000 years younger than previously thought.
So yes, it has and is being cross referenced with tree-ring methods, and according to this article, the new tree-ring research suggests the artifacts to be older than previously thought
That being said, the article further explains the need to cross-reference carbon dating with other methods, as carbon dating assumes the amounts of carbon in the atmosphere has always been consistent, but more recently with fossil fuels, it hasn't.
In their words:
The basis of radiocarbon dating is simple: all living things absorb carbon from the atmosphere and food sources around them, including a certain amount of natural, radioactive carbon-14. When the plant or animal dies, they stop absorbing, but the radioactive carbon that they’ve accumulated continues to decay. Measuring the amount left over gives an estimate as to how long something has been dead.
But this basic calculation assumes that the amount of carbon-14 in the environment has been constant in time and space — which it hasn’t. In recent decades, the burning of fossil fuel and tests of nuclear bombs have radically altered the amount of carbon-14 in the air, and there are non-anthropogenic wobbles going much further back. During planetary magnetic-field reversals, for example, more solar radiation enters the atmosphere, producing more carbon-14. The oceans also suck up carbon — a little more so in the Southern Hemisphere, where there is more ocean — and circulate it for centuries, further complicating things.
So they explain that other methods are needed to verify it. Although other answers on here have suggested tree ring evidence explicitly, the article says that more recently there have been other methods as well:
Since the 1960s, researchers have mainly done this recalibration with trees, counting annual rings to get calendar dates and matching those with measured radiocarbon dates. The oldest single tree for which this has been done, a bristlecone pine from California, was about 5,000 years old. By matching up the relative widths of rings from one tree to another, including from bogs and historic buildings, the tree record has now been pushed back to 13,910 years ago.
World's largest hoard of carbon dates goes global
Since 1998 there have been four official IntCal calibrations, adding in data from laminated lake and marine sediments, cave stalagmites and corals (which can be both radiocarbon dated and independently assessed using techniques such as radioactive thorium/uranium dating). In 2018, some stalagmites in Hulu Cave in China provided a datable record stretching back 54,000 years 1.
That footnote 1 in the article reads "Cheng, H. et al. Science 362, 1293–1297" which is quoted in sciencemag.org. As they say there:
An accurate, precise record of the carbon-14 (14C) content of the atmosphere is important for developing chronologies in climate change, archaeology, and many other disciplines. Cheng et al. provide a record that covers the full range of the 14C dating method (∼54,000 years), using paired measurements of 14C/12C and thorium-230 (230Th) ages from two stalagmites from Hulu Cave, China. The advantage of matching absolute 230Th ages and 14C/12C allowed the authors to fashion a seamless record from a single source with low uncertainties, particularly in the older sections.
So besides for tree ring dating, they also use the "thorium-230Th" method, which is slightly different than Carbon-12 and Carbon-14 methods.
They explain more in the article the necessities of cross referencing carbon 12-14 models with others (not necessarily tree ring models), in their words:
Libby pioneered the 14C dating method (1), which revolutionized a number of scientific disciplines, most notably archeology and climatology. However, variations in atmospheric 14C, likely caused by changes in the shielding of cosmic rays induced by the Earth’s and Sun’s magnetic fields and/or the redistribution of 14C among different carbon reservoirs, were soon recognized (2). These changes necessitate the calibration of 14C ages against a calendar time scale. A precise and accurate 14C calibration is considered the Holy Grail of radiocarbon dating.
Our ability to calibrate the 14C time scale has been limited by our ability to establish the absolute age of a material that contains information about atmospheric 14C/12C. By the late 1980s, the most recent portion of the 14C time scale [last ~10 thousand years (ka)] was calibrated extremely precisely using dendrochronology. The development of mass spectrometric 230Th dating methods (3) and their continued refinement (4) opened up the possibility of extending the calibration much deeper in time, led to the first large extension of the calibration well back into the Pleistocene (5), and ultimately has led to the current contribution. However, the 230Th dating approach has its own constraints. Corals, which are good materials for 230Th dating, do not accumulate continuously over thousands of years and are difficult to collect since those in the time range of interest are now largely submerged. Stalagmites, which can be excellent choices for 230Th dating, typically contain a significant fraction of carbon ultimately derived from limestone bedrock, which is essentially 14C-free. Stalagmite-based calibrations must therefore correct for a dead carbon fraction (DCF), which can be large and variable and is typically the main hurdle in such efforts (6, 7).
Southon et al. (8) demonstrated that the DCF in one Hulu Cave (32°30ʹN, 119°10ʹE) stalagmite, H82, was unusually small and stable, allowing a precise and accurate 14C calibration in the 26.8 to 10.6 ka B.P. (before the present; “present” is 1950 CE) interval (fig. S1). Here, we show that older Hulu Cave stalagmites, MSD and MSL, have similarly low and stable DCFs (Figs. 1 and 2), which allow for precise and accurate 14C calibration for the remainder of the 14C time scale back to ~54 ka B.P.
So they mention the 230Th method, but explain how that alone also has various inconsistencies, since the materials they collect it from, such as coral, have not been demonstrated to take it in consistently for thousands of years
But besides for that various sets of data (using the four methods mention there) from a cave in China seem to show similar, yet slightly different results. Graph
Basically, based on the data from the Hulu cave from the other methods, they attempt to put together the relative ages and resolve the previous uncertainties they had:
Considering the full record, there is a general correspondence with the latest IntCal compilation (17) (Figs. 1 and 2) within fairly large uncertainties, confirming the general validity of the compilation. However, for the portion older than 30 ka B.P., clear differences emerge. The Hulu record has less uncertainty and resolves previously unknown fine-scale structure. Between 33.5 and 42.5 ka B.P., the Hulu record indicates larger offsets between 230Th ages and 14C ages than IntCal13, with offsets between the records as high as 1 ka, corresponding to a higher Δ14C by as much as 170‰ as recorded at Hulu. Conversely, from 42.5 ka B.P. to the end of the IntCal curve at 50 ka B.P., **the Hulu record indicates smaller offsets between 230Th and 14C ages, by ~1 ka, which corresponds to ~140‰ lower Δ14C. **From 50 to 54 ka B.P., the Hulu curve indicates similar though nominally higher Δ14C than during the subsequent few millennia. Another notable difference is the sharper and higher amplitude increase in Δ14C around 42.5 ka B.P. A notable similarity is the lack of a prominent low Δ14C excursion around 31 ka B.P. This low, present in Cariaco sediment and Bahamas speleothem datasets (7, 18), was omitted from IntCal13 because of its absence from the Lake Suigetsu record (19). The Hulu data support this omission.
They then explain some of the irregularities with the other methods and 14C alone, and conclude they usually need all of them together to get a somewhat consistent picture:
14C ages are generally less than calendar ages throughout the full record, reaching a maximum offset of ~5200 years between ~39.3 and ~40.8 ka B.P. (Fig. 1).** The offset is largely due to higher atmospheric Δ14C, although there is also a progressive offset of 2.83% of the age due to the use of the Libby half-life in calculating the 14C age. **Between 54 and 43 ka B.P., **Δ14C values range between 0 and 300‰, then increase sharply to values exceeding 600‰ by 42 ka B.P. **(Fig. 2). High values continue until 38.8 ka B.P., reaching the highest values in the full record of 700‰ at 40.8 and 39.3 ka B.P. Between 38.8 and 38.0 ka B.P., Δ14C decreases sharply to values around 500%. Between 38.0 and 25.0 ka B.P., Δ14C values exhibit millennial-scale variability with highs around 600% and lows around 400%. Notable is a relative high of about 600% at 33.8 ka B.P. From 25.0 ka B.P. to the mid-19th century (as previously known), Δ14C values gradually diminish from around 500% to 0, with significant changes in slope between 16 and 11 ka B.P.
The new data provide critical constraints on the causes of changes in Δ14C during the last 54 ka. The millennial-scale pattern of Δ14C variations (Fig. 3) has similarities to the geomagnetic record (Virtual Axial Dipole Moment data) (20), suggesting that changes in shielding of cosmic rays by the geomagnetic field are responsible for much of the millennial-scale variation in Δ14C. Of note is the coincidence within tight age uncertainties between the abrupt increase in Hulu Δ14C and the onset of the Laschamp magnetic excursion at ~42.3 ka B.P. (21), as well as between the period of weakest geomagnetic field during the Laschamp (~41.1 ka B.P.) (21), which correlates with the highest Δ14C values over the past 54 ka. This suggests that the Laschamp is responsible for both of these features. Additionally, a second prominent peak in the Hulu record at ~34 ka B.P. is consistent with the timing of the Mono Lake excursion (22), suggesting that this excursion is responsible for the Δ14C peak (Fig. 3).
They then provide a chart showing the different ages and dates arrived at using the 4 different methods.
They explain possibilities for the inconsistencies:
The broad lowering of Δ14C throughout this interval **could plausibly result from progressively increasing ocean ventilation. **All other factors being equal, the shorter the mixing time, the less time for 14C to decay, the more 14C in deep waters and, by mass balance, the lower the Δ14C of the atmosphere. Presuming an average deep water age of 1000 years at 11 ka B.P. and a 60:1 ratio of deep water to atmospheric carbon, the lowering of atmospheric Δ14C over this time period can be explained by a progressive shift in deep water age from about 3000 years at 25 ka B.P. to the assumed 1000-year value at 11 ka B.P.
They conclude that they are still working on verifying it:
There is some support for the inference of increasing ventilation with time, as observations indicate that the deep Southern Ocean and South Pacific were poorly ventilated at the last glacial maximum (32–34). Deep ocean Δ14C data for times since the last glacial maximum (35) do not clearly resolve pre-Holocene from Holocene ventilation ages, but they also do not preclude large pre-Holocene ventilation ages. Thus, while it is likely that deep ocean ventilation change accounts for a portion of the residual 25 to 11 ka B.P. Δ14C drop, it is still not clear whether it can account for the full drop. Further work is needed to close the loop on this critical issue.
So it would seem from that article that there are four general methods they use, which give slightly different results (that they are still working to resolve) which are
- Δ14C residual "Hulu-model" which itself is divided into
a) "Dust flux" (mg m-2 yr-1) and
b) CO2 (ppmv)
- Δ14C "residual-detrended" hulu model
- Hulu 18O (VPDB) and
- NGRIP 18O (SMOW)
as referenced in the above linked image and explained in the article, as well as this image which includes the tree ring method
and explained in the beginning:
Fig. 1 Hulu speleothem 14C versus 230Th ages and comparison between Hulu and IntCal13 14C ages.
(A) Hulu [olive-brown, H82 (8); blue, MSD, and green, MSL (this study), and IntCal13 14C (17)] vs. 230Th ages. 14C error bars are 1σ. For clarity, uncertainties in IntCal13 are not shown. The floating tree ring Δ14C datasets (purple) (14, 15) are tuned to the Hulu 14C record (11). The red square (1σ) is the independent data point based on 14C measurements on wood associated with the Ar-Ar dated Campanian Ignimbrite (13). (B) 14C age difference (black) between Hulu dataset and IntCal13 (17). The gray envelope shows the uncertainty (1σ). Hulu 14C ages are corrected for the DCF (450 ± 70 years) (8). (C) Calendar age minus IntCal13 (red)/Hulu (blue) 14C age. The light blue envelope shows the uncertainty (1σ). The three Hulu sample datasets replicate over contemporary growth periods. Hulu Cave 14C data are consistent with IntCal13 between ~10.6 and 33.3 ka B.P. but lower in 14C ages between ~33.3 and 42 ka B.P. and higher between 42 and 53 ka B.P.
As well as Figure 2 that they explain:
Fig. 2 Comparison of Hulu Δ14C data with IntCal13.
Hulu Δ14C data are shown with error bars with the same color codes as in Fig. 1. IntCal13 and its dataset (17) are shown in the gray envelope and gray bars. 14C error bars are 1σ. Hulu data overlap with IntCal13 between ~10.6 and 33.3 ka B.P.; however, there are substantial offsets, particularly before 30 ka B.P., and the Hulu record exhibits substantial previously unknown millennial-scale structure. The purple error bars and red square are the floating tree ring series and Campanian Ignimbrite data, as in Fig. 1.
So there are several uncertainties with these methods and much of it is still being worked on, but even if there weren't the young earth creationists will just tell you that the trees were created to look old in the first place, similarly to how a miraculous flood would have altered the carbon-14 data, so even if the other methods were consistent, that wouldn't disprove anything to them, as the question seems to suggest it would.